Legendre Polynomial Rodrigues Formula Proof Of Rodrigues Form

Scilab Tutorial Rodrigue S Formula Of Legendre Polynomials Scilab 3. (the general formula of legendre polynomial s is given by following equation: pk(x) = k 2 k − 1 2 ∑ m = 0 ( − 1)m(2k − 2m)! 2km!(k − m)! 1 (k − 2m)!xk − 2m. the rodrigues' formula is: 1 2kk! dk dxk[(x2 − 1)k] the binomial theorem is as follow: (x y)k = k ∑ i = 0 k! i!(k − i)!xk − iyi. then (x2 − 1)k = k ∑ i = 0 k. Legendre. legendre polynomials: rodriques’ formula and recursion relations. jackson says “by manipulation of the power series solutions it is possible to obtain a compact representation of the legendre polynomials known as rodrigues’ formula.”. here is a proof that rodrigues’ formula indeed produces a solution to legendre’s.

Rodrigues Formula Legendre Equation Rodrigues Formula Proofођ In mathematics, rodrigues' formula (formerly called the ivory–jacobi formula) generates the legendre polynomials. it was independently introduced by olinde rodrigues ( 1816 ), sir james ivory ( 1824) and carl gustav jacobi ( 1827 ). the name "rodrigues formula" was introduced by heine in 1878, after hermite pointed out in 1865 that rodrigues. A second proof of the three term recursion formula can be obtained from the generating function of the legendre polynomials. many special functions have such generating functions . in this case it is given by. Where the polynomials form the basis of the associated legendre functions, which in turn form part of the solution of the three dimensional schrödinger equation. we’ll run through the proof here. using the rodrigues formula, we have z 1 1 p m(x)p n(x)dx= 1 2m nm!n! z 1 1 dm dxm (x2 1)m dn dxn (x2 1)ndx (9). This page titled 11.2: properties of legendre polynomials is shared under a cc by nc sa 2.0 license and was authored, remixed, and or curated by niels walet via source content that was edited to the style and standards of the libretexts platform.
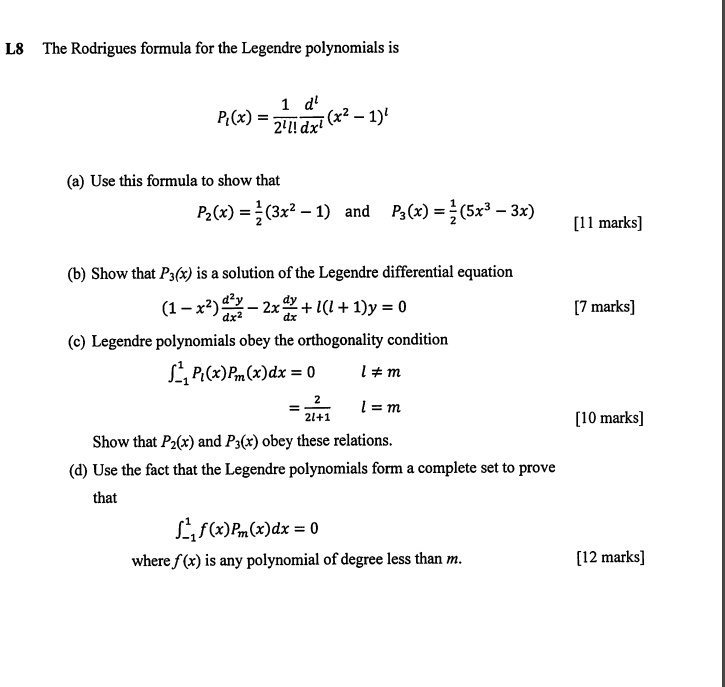
Solved L8 The Rodrigues Formula For The Legendre Polynomials Is P X Where the polynomials form the basis of the associated legendre functions, which in turn form part of the solution of the three dimensional schrödinger equation. we’ll run through the proof here. using the rodrigues formula, we have z 1 1 p m(x)p n(x)dx= 1 2m nm!n! z 1 1 dm dxm (x2 1)m dn dxn (x2 1)ndx (9). This page titled 11.2: properties of legendre polynomials is shared under a cc by nc sa 2.0 license and was authored, remixed, and or curated by niels walet via source content that was edited to the style and standards of the libretexts platform. 1 adrien marie legendre (1752 1833) was a french mathematician who made many contributions to analysis and algebra. (1. in example 4.4 we found that for n an integer, there are polynomial solutions. the first of these are given by p0(x) = c0, p1(x) = c1x, and p2(x) = c2(1 − 3x2). We showed that pn(x), the legendre polynomial of degree n, satisfies rodrigues’s formula: pn(x) = 1 2nn! dn dxn ¡ (x2 −1)n ¢, n = 0,1,2, , provided the following lemma is proved. lemma the polynomial pn(x) = d n dxn ¡ (x2 −1)n ¢ (n = 1,2, ) is orthogonal to any polynomial of degree less than n. proof. let q(x) be a polynomial of.

14 Rodrigue S Formula For Legendre S Function Of First Kind Legendre 1 adrien marie legendre (1752 1833) was a french mathematician who made many contributions to analysis and algebra. (1. in example 4.4 we found that for n an integer, there are polynomial solutions. the first of these are given by p0(x) = c0, p1(x) = c1x, and p2(x) = c2(1 − 3x2). We showed that pn(x), the legendre polynomial of degree n, satisfies rodrigues’s formula: pn(x) = 1 2nn! dn dxn ¡ (x2 −1)n ¢, n = 0,1,2, , provided the following lemma is proved. lemma the polynomial pn(x) = d n dxn ¡ (x2 −1)n ¢ (n = 1,2, ) is orthogonal to any polynomial of degree less than n. proof. let q(x) be a polynomial of.

Rodrigues Formula Legendre Polynomial Proof Of Rodrigues Form

Comments are closed.